by C.R. (Gus) Manning, PhD, CIH, FAIHA
We regularly receive questions from clients who are interested in the level of uncertainty in their badge results. While the short answer is simply that the results meet the OSHA requirements, there are actually several components which go into determining the sampling and analytical uncertainty for any monitoring method.
Understanding Accuracy & Uncertainty
To resolve the confusion in conversations about accuracy and precision, today’s standard-setters prefer to talk about uncertainty, which allows us to combine accuracy and precision into a single statement with a rigorous definition. Uncertainty is best understood as the probability of a measured value being within a stated range of the true value.
When we report a test result of an exposure level at 48 parts per million (ppm), due to natural variations, we know the true value can only be known within a range of values. Based on the statistical results of studies using standard preparations, we can estimate the probability (likelihood) of the true value being within a certain range of the reported value.
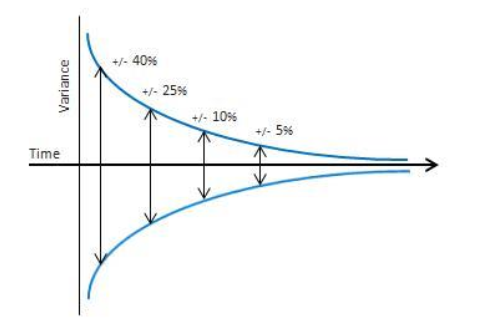
Since quality control and industrial hygiene professionals want to be pretty sure of decisions, 95% is taken as a popular “level of confidence”. To have 95% confidence means you believe that 95 out of 100 (i.e. 19 out of 20) tests on an identical sample will fall within a stated range.
When data variation is typical or random (what statisticians call “normal”), 95% of values in the data population will fall within two times the standard deviation. This means that you can run a series of replicate tests using standard (known) preparations and calculate the standard deviation to estimate the range of values that 95% of a population will fall within.
Uncertainty in Sampling & Analysis vs Uncertainty in Exposures
In the real world, Uncertainty in Exposure Levels is great due to natural workplace variation (concentration variations at different locations and times in a workplace). Rappaport (a), Nicas (b), and others demonstrated that workplace variation is typically more than 5-10 times as great as the typical variation (uncertainty) in sampling and analytical methods. Thus, in its AIHA Exposure Decision Analysis (EDA) system, AIHA authors ignore the uncertainty in sampling & analysis as being “negligible compared to the uncertainty in workplace exposure levels” (provided sampling & analysis methods comply with OSHA/NIOSH guidelines).
(a) S. M. Rappaport, H. Kromhouta & E. Symanski (1993) Variation of Exposure between Workers in Homogeneous Exposure Groups, AIHA Journal, 54(11), 654-662.
(b) Nicas, M., Simmons, B. P., & Spear, R. C. (1991). Environmental Versus Analytical Variability in Exposure Measurements, AIHA Journal, 52(12), 553–557.
Desirable Uncertainty for IH Sampling & Analysis
Among industrial and occupational hygienists around the world (including NIOSH and OSHA), the desired range of uncertainty is most commonly taken as ± 25% of the measured value. That is, IH experts would like each test result from a sampling and analytical method to give a result that will be within ± 25% of the true value in 95% of trials.
Uncertainty in Sampling versus Analysis
So, industrial hygienists have a desired uncertainty of ± 25% at a 95% confidence level for sampling and analysis, but the overall uncertainty arises from uncertainty in each step of the process. Thus, you would expect a portion of uncertainty to arise from sampling while a separate additional portion would arise from the analysis. In practice it is difficult to separate these portions, but our process for doing so is described in the following paragraphs.
Estimated Uncertainty in Lab Analysis
Laboratories try to estimate the Uncertainty in Lab Analysis by preparing so-called “spikes” (standard samples inoculated with known amounts of analyte), and then recovering (analyzing) the spiked samples. Spike & recovery methods seek to avoid the uncertainty due to workplace variation and the sampling process by preparing replicates with known amounts of analyte added to each sampler. While sample spikes have variation (uncertainty), each spike has much less variation than that associated with workplace uncertainty or sampling uncertainty.
Accordingly, lab analysis uncertainty is typically determined by spike & recovery using standard preparations inoculated with known analyte amounts. Assuming natural variations are random, two times the standard deviation (2.0 x RSD) of the replicate data from known (identical) spikes is taken to represent the range of values within the 95% confidence level. Studies at Assay Technology have demonstrated an RSD of ± 3-8% across a range of analytes which equates to an uncertainty of ± 6-16%, depending on the analyte.
Estimated Uncertainty in Sampling
While sampling uncertainty can be avoided by spike & recovery, it is impossible to acquire sampling data without also performing lab analysis, so the uncertainty of lab analysis is usually mixed in with sampling uncertainty. To remove the lab analysis uncertainty, it is necessary to determine the uncertainty associated with sampling and analysis, and then subtract the uncertainty attributed to lab analysis alone.
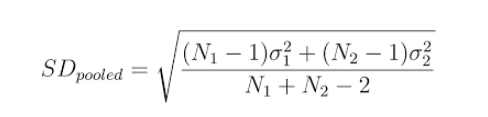
The Uncertainty of Sampling is estimated from the pooled RSD (Relative Standard Deviation) for multiple sets of replicate samplers exposed to real-world vapor spikes (exposures) within several independent vapor spike chamber runs. Data across a range of analytes and personal monitoring badges has demonstrated an RSD of ± 3-5%. Two times the pooled standard deviation of such data sets provides an estimate of the uncertainty of sampling at 95% confidence, or ± 6-10% of the result.
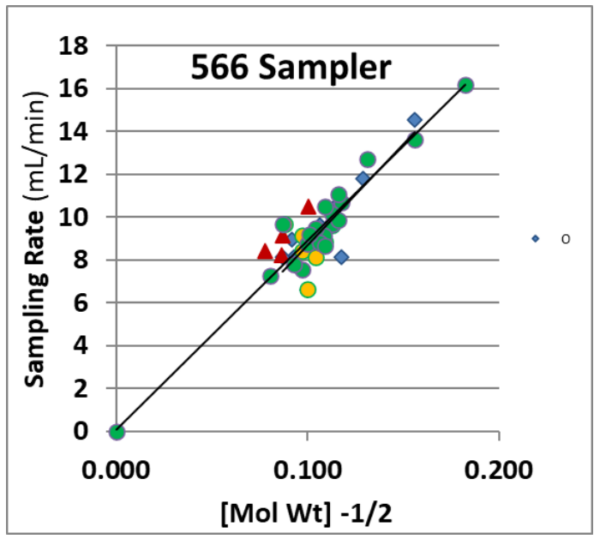
Estimated Uncertainty in Sampling Rate
A final level of Uncertainty of Sampling must include an estimate of the Uncertainty in knowing the Sampling Rate. Based on a summation of multiple statistical studies over multiple exposure chamber runs, we have estimated the RSD in sampling rate at ± 4% when the sampling rate is laboratory-determined and ± 8% when the sampling rate has been determined by semi-empirical extrapolation based on molecular properties.
